More control, better insights, less friction.
All of the familiar LMS workflows reimagined in a modern, efficient interface.
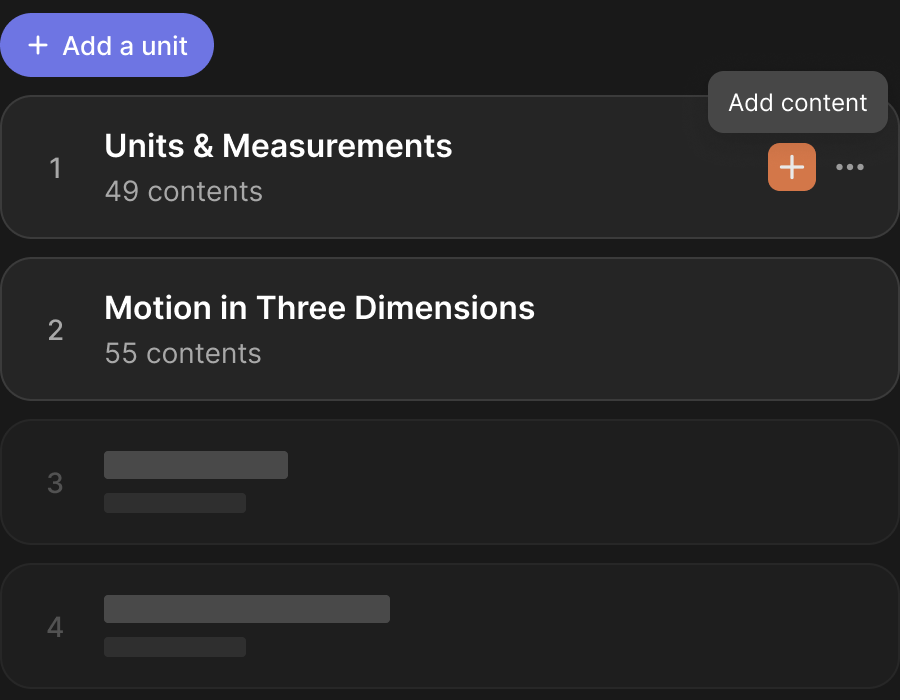
Rapid course creation tools
Build and update your courses effortlessly using simple tools that support text, images, videos, links, and interactive elements.
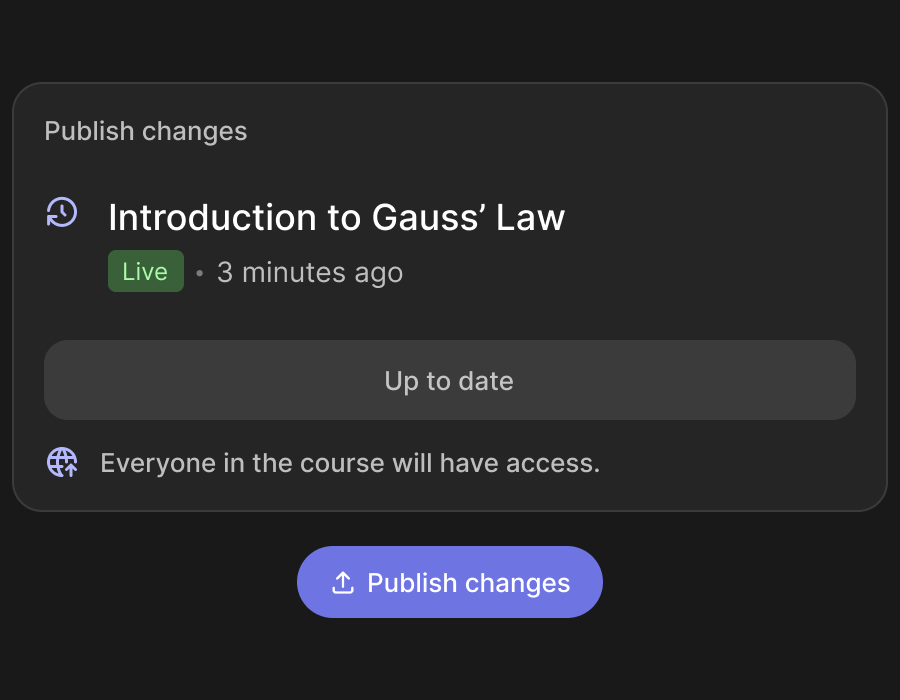
Content version control
Edit, improve, and iterate on your materials without risk. Embox can safely track and save changes across material content.
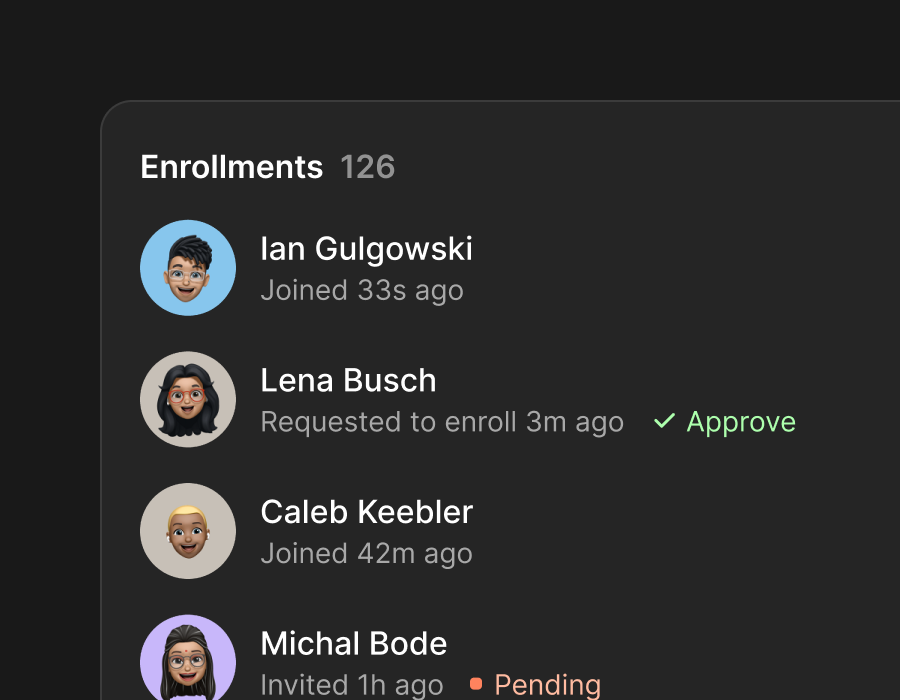
Powerful student management
Easily manage, track, and support your learners with tools for enrollment, progress monitoring, performance insights, and feedback communication.
Granular visibility settings
Control exactly what students see and when. Schedule content releases, hide drafts, or lock assessments until prerequisites are met.